Introduction to Financial Maths
expand_moreIntroduction to Financial Maths
Was this lesson helpful?
thumb_up thumb_downIntroduction to Financial Maths
- Financial maths is a branch of mathematics that deals with financial problems. particularly monetary value of things.
Monetary value
- Refers to the value of a product or service measured in terms of money. E.g., The bread costs approximately R20, meaning its monetary value is R20.
-
Monetary value is subject to change over time, which may be due to one of the following:
- Interest - Is the money charged when a debt is incurred through borrowing money, taking a car, phone, house, etc. on credit.
- Inflation - Inflation is the rate of increase in prices over a given period of time. for example, monetary value or price of bread in 2005 was R5, today in 2024 it is R20.
- Deflation - Deflation is the rate of decrease in prices over a given period of time. for example, monetary value or price of petrol was R23.01 in May 2023, and 22.79 in December 2023.
- Depreciation - Things lose value over time due to wear and tear or becoming outdated. for example, you buy a phone for R5000, after a year you want to sell it. The fair price should be below R5000 due to wear and tear.
- Appreciation - Things gain value over time due to inflation, market demand, or improvement to the asset. House prices usually increase there's not enough houses to house everybody, hence there is always a high demand for houses.
- Interest, Inflation, Deflation, Depreciation, and Appreciation can be represented as capital 'I'.
Rate of Change
- Percentage with which value changes over time, represented by small letter 'i' or r.
- Represents all monetetary changes, Interest rate, Inflation rate, Deflation rate, Depreciation rate, or Appreciation rate
- Expressed as a percentage. E.g., Interest rate of 7% or Inflation rate of 3%.
Two types of changes: Growth and Decay
- Simple growth and Decay: Constant growth or decrease
- Compound growth and Decay: Constant rate calculated on a different amount each year
Converting percentages to decimals
expand_moreConverting percentages to decimals
Converting percentages to decimals, When doing financial maths calculations, need to know how to convert percentages to decimals.
Was this lesson helpful?
thumb_up thumb_downSimple interest
expand_moreSimple interest
simple interest is the interest amount for a particular principal amount of money at some rate of interest.
Was this lesson helpful?
thumb_up thumb_downSimple Change in monetary value
- I is the total amount of monetary value change
- P is the initial monetary value of asset, or money borrowed or lended.
- i is the rate of change in percentage, from the principal each passing time, which is usually per year for simple rate of change. Note: rate of change can also be represented as r.
- t is the amount of time the rate of change counted apon. Note: duration can also be represented as n.
-
You deposit R2000 at Capitec bank for a 5% interest rate annual.
How much interest would you have earned after 5 years?
first divide the given interest rate by 100.
i = 5/100
i = 0.05
I = Pit
I = R2000 * 0.05 * 5
I = R500
Therefore, you would have earned R500 interest in your R2000 deposit.
Now, how much in total will be available in your account after those 5 years?
The total is usually represented by capital letter 'A'. Therefore, A is the sum of Principal and Interest earned.
A = P + I
A = R2000 + R500
A = R2500- You need to calculate the final amount available after a given duration without calculating interest earned first
A = P + I
I = Pit, hence you can replace I with Pit
A = P + Pit
Factorize, A = P (1 + it)
Equation: I = Pit, where:
0 years ago, Mr Ngubi decided to invest R0 in a bank account that paid simple interest at 0% p.a.
Calculate how much interest has Mr Gumbi earned over the 0 years .
Your answer into two decimal places.
Chances: 2
You get two chances for this question.
0 years ago, Mr Ngubi decided to invest R0 in a bank account that paid simple interest at 0% p.a.
What is the total amount does Mr Gumbi's account have after 0 years?
Chances: 3
You get three chances for this question.
- The first question you got 0/2
- The second question you got 0/3
Your total is therefore, 5/5.
Percentage
%
A Message here
Simple vs Compounded interest
expand_moreSimple vs Compounded interest
Was this lesson helpful?
thumb_up thumb_downSimple Interest
- The interest earned is equal to the principal times the rate times the time. Meaning, the principal never changes, the interest is always counted using the initial investment or principle. Hence, also interest earned in a given timeline remains the same.
-
You deposit R2000 at Capitec bank for a 5% interest rate annual.
first divide the given interest rate by 100.
i = 5/100
i = 0.05
first year interest
I = Pit
I = R2000 * 0.05 * 1
I = R100
second year interest
I = Pit
I = R2000 * 0.05 * 1
I = R100
third year interest
I = Pit
I = R2000 * 0.05 * 1
I = R100
Compound Interest
- In contrast to Simple Interest, Compound interest The interest earned each timeline (yearly, Quarterly, Monthly, Weekly, daily, hourly) is added to the principal for the next timeline's calculation.
- You deposit R2000 at Capitec bank compounded at 5% annual.
first divide the given interest rate by 100.
i = 5/100
i = 0.05
first year interest
I = Pit
I = R2000 * 0.05 * 1
I = R100
second year interest
The new principal is R2100, because the previous timeline earned interest get added to the initial principal (R2000 + R100 = R2100). I = Pit
I = R2100 * 0.05 * 1
I = R105
third year interest
The new principal is R2250, because R2100 + R105 = R2250. I = Pit
I = R2250 * 0.05 * 1
I = R110,25
- Therefore in the period of three years, you will earn R300 with a total of R2300 in your account if your R2000 deposit is 5% Simple or earn R312,25 interest and total amount of R2315,25 in your account if compounded.
Compound Interest - The Formula (Compounded Yearly)
expand_moreCompound Interest - The Formula (Compounded Yearly)
Was this lesson helpful?
thumb_up thumb_downCompound Interest Formula
- The accumulated amount (A) is calculated using the formula:
-
A = P(1 + r/m)mt
Where:- A = accumulated amount
- P = principal invested
- r = interest rate
- m = number of conversions per year
- t = number of years
- You deposit R2000 at Capitec bank compounded at 5% annual.
Find the accumulated amount in 3 years
first divide the given interest rate by 100.
i = 5/100
i = 0.05
A = P(1 + r/m)mt
A = R2000(1 + 0.05/1)(1)(3)
A = R2000(1 + 0.05)3
A = R2000(1.05)3
A = R2000(1.1025)
A = R2315,25
Compound Interest - Problem Scenarios
expand_moreCompound Interest - Problem Scenarios
Compound Interest questions can come in many ways, this video demonstrate some of the possible scenarios.
Was this lesson helpful?
thumb_up thumb_down 0 years ago, Njabula decided to invest R0 in a bank account that paid simple interest at 0% p.a
How much will he have in his account after 0 years .
Your answer into two decimal places.
Chances: 2
You get two chances for this question.
0 years ago, Njabula decided to invest R0 in a bank account that paid simple interest at 0% p.a
How much will he have in his account after 0 years, if the interest was compounded?
Your answer into two decimal places.
Chances: 2
You get two chances for this question.
Boitumelo have R0 in her saving's account after investing R0, 0 years ago compounded quarterly.
What was the interest rate of boitumelo's savings account?
Your answer into two decimal places.
Chances: 4
You get a maximum of four chances for this question.
After those 0 years, the interest rate was changed to 0% compounded monthly.
How long will it take for Boitumelo's account to have R0?
Roundoff your answer to a whole number.
Chances: 4
You get a maximum of four chances for this question.
- The first question you got 0/2
- The second question you got 0/2
- The third question you got 0/4
- The forth question you got 0/4
Your total is therefore, 0/12.
Percentage
%
A Message here
Effective Rate of Interest
expand_moreEffective Rate of Interest
The effective rate of interest refers to the equivalent rate of interest needed to earn the same amount of money when the money is invested at simple interest, as if it were compounded monthly or daily.
Was this lesson helpful?
thumb_up thumb_downEffective Rate of Interest
- It calculates the rate required for the same earnings with different compounding frequencies.
Calculation for Monthly Compounding
- reff = (1 + rnom / m)m - 1 Where:
reff = is the effective rate of interest.
rnom = is the nominal rate of interest.
m = is the number of conversions per year. - m = 12 because there are 12 months in a year
- reff = (1 + rnom / m)m - 1
reff = (1 + 0.05 / 12)12 - 1
reff = 0.05116 * 100
reff = 5.116%
Example: For R1000 invested at 5% with monthly compounding:
Calculation daily Compounding
- m = 365, because there are 365 days in a year.
- reff = (1 + rnom / m)m - 1
reff = (1 + 0.05 / 365)365 - 1
reff = 0.05127 * 100
reff = 5.127%
Comparison of Monthly and Daily Compounding
- The effective rate of interest for daily compounding is slightly higher at 5.127% compared to 5.116% for monthly compounding.
- This means the equivalent rate needed to earn the same amount of money is higher for daily compounding.
Time Value of Money - Present Value vs Future Value
expand_moreTime Value of Money - Present Value vs Future Value
Future value represents the worth of a current asset, investment, or cash flow at a specific date in the future based on an assumed rate of growth.
Was this lesson helpful?
thumb_up thumb_downFuture Value and Present Value
- Future value (FV) represents the value of money in the future, while present value (PV) represents the value of money today.
- Find the future value of $10,000 in 20 years at an annual interest rate of 6%
- It is almost exactly the same as the formula for compound interest, the only difference is that A is FV and P is PV.
- FV = PV * (1 + r)n
- m = number of conversions per year
- t = number of years
Future value/Present value Formula
- The future value of $10,000 in 20 years at 6% interest is $32,071.35.
- Present value is the value of an amount of money at the current time
- Present value of $100,000 in 10 years at an annual interest rate of 6% is calculated using the formula: PV = FV / (1 + r)n.
- The present value of $100,000 in 10 years at 6% interest is $55,839.48.
- Illustrates the time value of money, where money is worth more now than in the future.
- Purchasing power decreases as time progresses.
- The same amount of money is worth more now than in the future.
DEPRECIATION using the Reducing Balance Method
expand_moreDEPRECIATION using the Reducing Balance Method
Depreciation is the loss of the value of an asset over a period of time. for example, your bought your phone for R5000 three years ago, today its value is propably R1500 - R2000 because of wear and tear, or obselescence.
Was this lesson helpful?
thumb_up thumb_downDepreciation and Reducing Balance Method
- Depreciation is the loss of the value of an asset over a period of time.
Example Calculation
- Anthony bought a delivery vehicle for $80,000 with an estimated useful life of three years at a 30% rate.
- Year 1: Depreciation = $80,000 - 30% = $24,000.
- Year 2: Depreciation = $80,000 - ($24,000 + 30% of $80,000) = $16,800.
- Year 3: Depreciation = $80,000 - ($24,000 + $16,800) - 30% = $11,760.
- Overall Depreciation in those three years is therefore, the sum of all Depreciation.
Overall Depreciation = Year 1: Depreciation + Year 2: Depreciation + Year 2: Depreciation
Overall Depreciation = Year 1: $24000 + $16800 + 11760
Overall Depreciation = $52560
Book Value
- Net book value represents the present value of the asset in financial statements.
- And is calculated by substracting the overall depreciation by original price of the asset.
- E.g., Net book value of Anthony's vehicle after 3 years = $80000 - $52560
Net book value = $27440 - Or you can use the fornula, A = P(1 - r)n
- A = $80000(1 - 0.3)3
- A = $27440
Conclusion
- The reducing balance method results in higher depreciation in the first year, gradually reducing over time.
The nominal interest rate of an investement is 0% per annum, compounded monthly.
Calculate the effective interest rate.
Your answer into two decimal places.
Chances: 2
You get a maximum of two chances for this question.
Calculate the effective interest rate if it was compounded weekly instead of monthly.
Your answer into two decimal places.
Chances: 2
You get a maximum of two chances for this question.
The current value of a property is R0
Calculate the property value in 0 years if the average annual property rate was 0%.
Your answer into two decimal places.
Chances: 3
You get a maximum of three chances for this question.
Andani bought a smartphone for R0 in 0.
Calculate the book value of the phone today if it depreciates at 0 p.a., according to the reducing balance method.
Chances: 3
You get a maximum of three chances for this question.
How much value has the phone lost over the years?
Chances: 2
You get a maximum of two chances for this question.
- The first question you got 0/2
- The second question you got 0/2
- The third question you got 0/3
- The forth question you got 0/3
- The fifth question you got 0/2
Your total is therefore, 0/6.
Percentage
%
A Message here
Solve the following:
0/0 ÷ 0/0
Chances: 1
You get one chance for this question.
Solve the following:
0/0 + (0)/0
Chances: 2
You get a maximum of two chances for this question.
Solve the following:
0/0 + 0/0
Chances: 2
You get a maximum of two chances for this question.
Solve the following:
0/0 - 0/0
Chances: 2
You get a maximum of two chances for this question.
A a box with a mass of 0kg rests on a flat surface, find the normal acting on the box.
Chances: 2
You get a maximum of four chances for this question.
Find the static friction of the box, if its coefficient was 0.

Chances: 2
You get a maximum of two chances for this question.
The box is now pushed horizontal to the right by a force of 0N is moving to the right with an acceleration of 0m/s2, what is the coefficient of the kinetic friction?
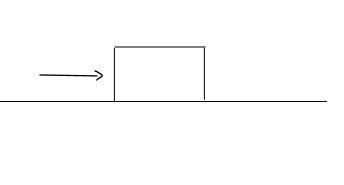
Chances: 3
You get a maximum of three chances for this question.
The box is now pushed at angle of 0° to the right by a force of 0N, Calculate the new normal force.
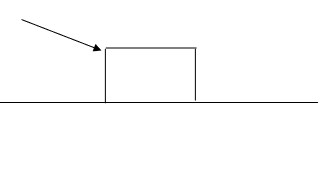
Chances: 3
You get a maximum of three chances for this question.
As the box continue to move, what is the acceleration of the box if kinetic friction coeffient was 0.

Chances: 3
You get a maximum of three chances for this question.